I completed quite a few sculpture projects in Spring 2006, most of which are pictured below.
While having dinner with Helaman Ferguson last Christmas at The CheeseCake Factory in Maryland, Helaman amused us by taking three paper napkins and twisting them together into a remarkably symmetric sculpture based on the three linked Borromean rings. I was inspired to try making one a bit larger in bronze. It was not so difficult to make the wax model, but the bronze casting has been difficult to polish. Here are pictures of the original paper model, and two views of the bronze casting, which has not been completely polished yet.




A second sculpture based on the same idea with just a single twist was cast on March 3, 2006. Because of the curved bottom, it is a bit unstable standing up, so it will need a special base to sit on, not shown.


Another bronze casting project I completed was a tetrahedron whose surfaces were concavely cuved inwards, and whose edges were twisted. I made two more versions of this sculpture, one with a pair of holes connecting two pairs of opposite faces, and another with the entire center empty, leaving only the framework of the curved edges and a suggestion of the faces.









The third bronze piece cast on March 3, 2006, was based on a torus (doughnut) but with six holes punched through, three punched radially out from the center, and three punched vertically perpendicular to the plane of the torus. I had carved the wax model so that many of the surfaces going between these six holes were saddle shaped (negatively curved). But since some parts of the torus remained uncarved, the result was not completely negatively curved. I plan to try more sculptures of this type, starting with a torus and removing pieces to create holes and saddle curves connecting them. It should be possible to make one which is like a Costa surface, completely negatively curved.



One sculpture made in an earlier casting had defects that required repair by welding. This one was related to the two twisted rings shown on another webpage, but consists of two connected rings, one is twisted many times like a Mobius strip, but the other is twisted in a completely different way, like a torus which has been pulled into a handle shape. Together they look like a hand mirror, but with no glass in which to look.

Many years ago I soldered three triangles of thin metal rod together to make a light sculpture which looked like stop-motion pictures of a single triangle flying through space. It was not strong enough to hold together for long, so I decided to try using stronger welding techniques and thicker rods to remake it. Here are pictures of the results.

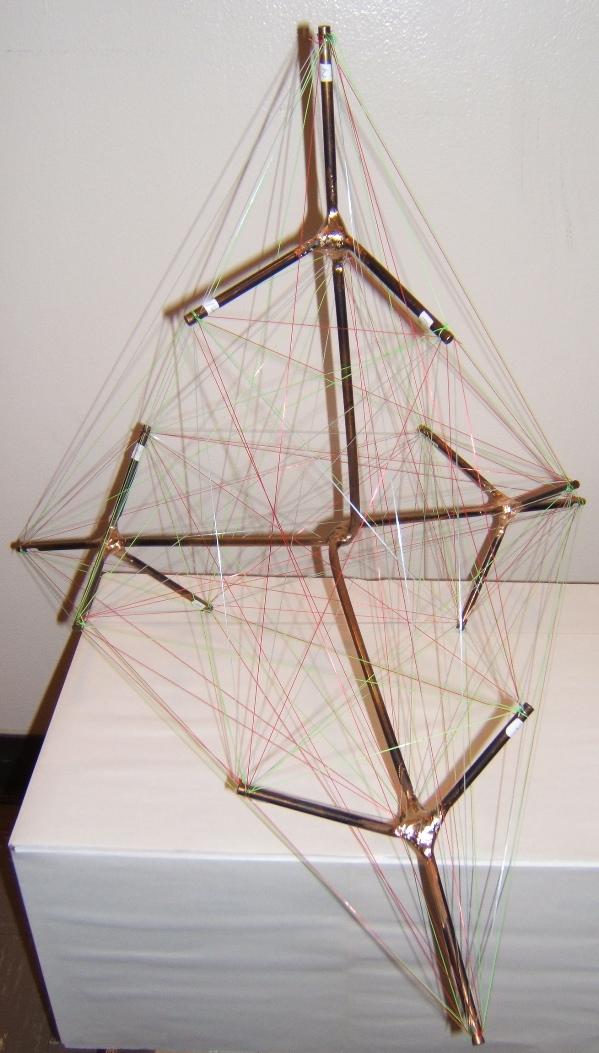
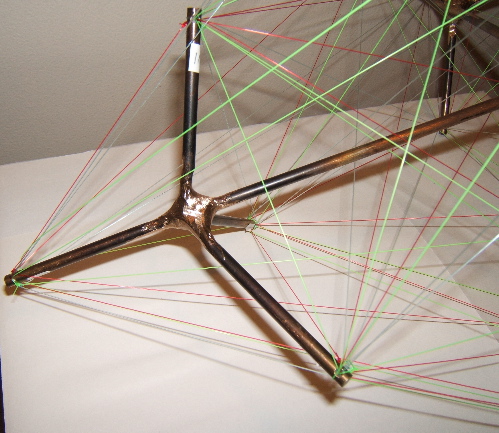
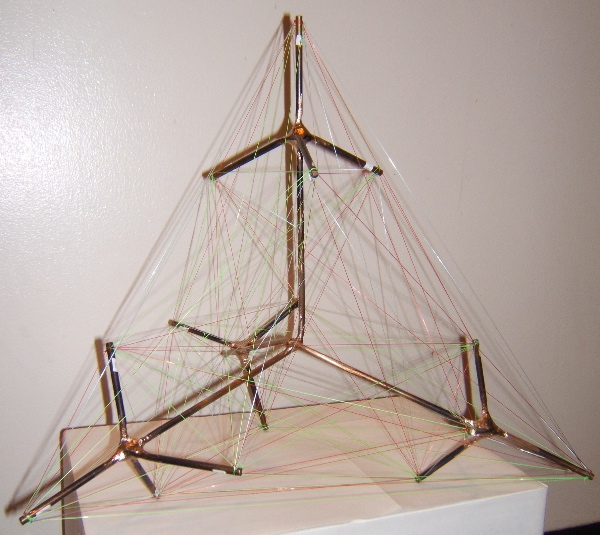
One of the most difficult and challenging sculpture projects I completed this semester was suggested to me by my colleague, Prof. Dikran Karagueuzian. There is a theorem in graph theory concerning the coloring of the complete graph on sixteen vertices, that three colors suffice to make a graph in which no triangle is monochromatic. With the help of the computer program, Maple, Dikran was able to find such a coloring and give explicit instructions on how to place the colored edges by threading colored plastic fishing line between the numbered vertices. My contribution to the construction was to weld a framework of 1/4" bronze rods into four tetrahedra, each placed at the ends of a larger tetrahedron, so that holes drilled into the ends of the smaller tetrahedra could be used as the sixteen vertices of the graph. I had to invent a special jig to hold the small rods in position at the precise angle (arccos(-1/2) = 109.74 degrees) to produce regular tetrahedra. The rods are actually the line segments from the geometric center of the tetrahedra to its four vertices. It is the plastic fishing line that becomes the edges of those tetrahedra, as well as all possible edges between pairs of vertices in different tetrahedra. It took approximately a month to produce the framework, and working together to thread the colored lines, Dikran and I took about three days to finish the sculpture. Pictures of the whole structure and detailed closeups of one tetrahedron are below. Dikran has hopes of having an expert glass blower create this amazing graph without a supporting frame! It was not easy positioning the tetrahedra so that there was a clear line of sight between each pair of vertices. In many cases the lines barely missed intersecting in space, so a more substantial material with thicker lines would need more careful placement to achieve sufficient clearance. I was very happy that my framework did achieve the result.


To see more types of sculpture I have tried, follow the following links:
Links back to:
Webpage of Alex Feingold,
Department of Mathematical Sciences,
Binghamton University.