Steel and Plastic Sculptures

by Alex J. Feingold

I have recently completed a sculpture inspired by my own research into a rank 3 hyperbolic Kac-Moody Lie algebra, where the root system is in a three dimensional space with an indefinite bilinear form. The real roots are all integral points on a single sheeted hyperboloid just outside the light cone of isotropic vectors, and the imaginary roots are all integral points on and inside that light cone. The integral points on the light cone can be described as lines through the origin corresponding to rational numbers and infinity. Each such line is the intersection of the cone with a tangent plane which also intersects the single sheeted hyperboloid in exactly two parallel lines. The integral points on those three parallel lines are the root system of an affine Kac-Moody Lie algebra g^ of type A_1^{(1)}. Thus, the geometry of those affine root systems shows the embedding of an infinite number of affine algebras g^ inside the rank 3 hyperbolic. This sculpture shows 60 such sets of parallel lines. It is one of the beautiful aspects of the curved surface of the hyperboloid that it can be built from straight lines. This piece is now owned by Craig Huneke, my good friend and classmate from graduate school at Yale.

Here are pictures of the single sheeted hyperboloid and cone.

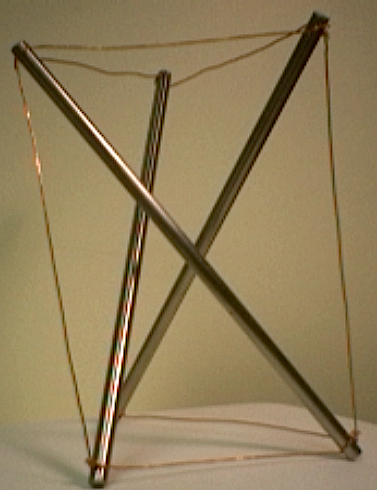
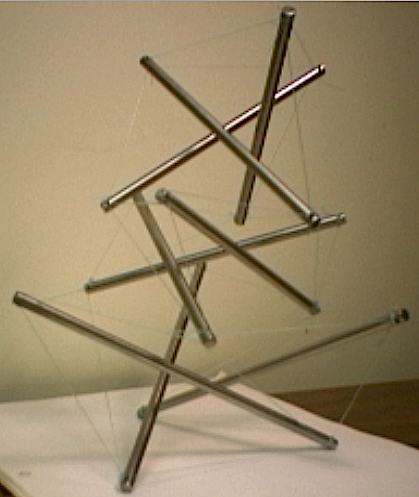
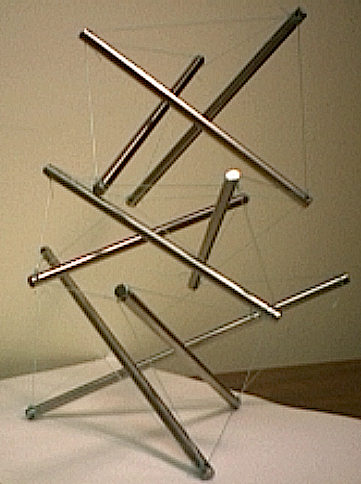
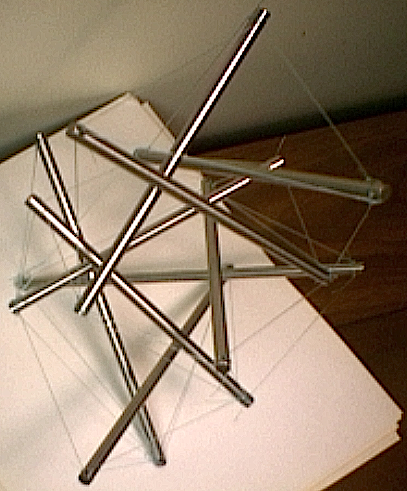
Here are pictures of tensegrity sculptures I made from stainless steel 1/4" rod left over from a kinetic sound sculpture. The rods are 8" on the bottom level, 6" on the other levels, and do not touch, but are held in a fixed position by the tensile strength of the wire (fishing line). This concept for sculpture was invented by Kenneth Snelson. One of the best examples of his work is the Needle Tower at the Hirschhorn Art Museum in Washington, DC, but his webpages are full of beautiful pictures of his wonderful structures. I hope he doesn't mind my imitating him.

After seeing a postcard with a picture of a sculpture of a Mobius band twisted into a trefoil knot made by the sculptor John Robinson, I decided to try to make one myself. But I wanted mine to rotate freely, supported in the air by a central pillar attached to a base plate polished enough to provide a reflection of the knot. In my first attempt I took a bar of steel and used an oxyaceteline torch to bend it into the desired shape. After two weeks, I had an approximation whose ends did not quite meet as required and which had some undesired bumps. I returned to the project later and managed to remove most of the bumps and got the ends to meet. After the ends were welded together by Kern Maass, I did some polishing with an edge grinder and other tools, but the base plate of stainless steel was too hard to polish to a mirror finish, so I put a plexiglass plate on top of it to provide some smooth reflection. The following pictures show the result, which could still be polished further. It makes a remarkable sound when hung by a wire and hit by a hammer, so perhaps it would do better as a mobile kinetic sound sculpture.

My second Mobius Trefoil Knot began with a wax model and was cast in silicon bronze. Although that project was completed in spring of 2006 on June 4, 2006, I am posting the pictures of it on this webpage for comparison with the steel trefoil knot shown above. It floats over a base plate of bronze polished to a mirror finish, balanced on a bronze rod so that it is free to rotate.

To see more types of sculpture I have tried, follow the following links:

Links back to:
Webpage of Alex Feingold,
Department of Mathematical Sciences,
Binghamton University.