On this page will be pictures of sculptures I made in Fall 2014. I have been working with a 3D graphics program, Rhino for Mac, to make a computer model of a figure 8 knot whose cross section is a triangle with concave sides (close to a hypocycloid) where the triangle rotates slightly as it goes around the curve so that when it comes back to the start, it has rotated just 120 degrees. That means there is only one edge and one surface, like a Mobius strip. I have made such sculptures in bronze before in a large size, most recently in Spring 2014, but my goal is to use 3D printing to make it accurately in a small size for jewelry. The first version I have just completed is the simpler one with no twisting, producing a figure 8 knot with concave cross section and no twisting, so there are three edges and three sides. A copy in stainless steel was printed by Shapeways.com, received on October 20, 2014, and is shown in the next two pictures.


The next two pictures show a silver version of the same untwisted figure 8 knot, received from Shapeways.com on Oct. 30, 2014. The difference in color is only because of the lighting.


The next three pictures show a polished bronze version of the twisted figure 8 knot, so I finally have the 1-sided Mobius figure 8 knot, received from Shapeways.com on Nov. 12, 2014. The differences in color are only because of the lighting.



The next picture shows a large (about 5.5" in the longest direction) white plastic version of the 1-sided Mobius figure 8 knot, received from Shapeways.com on Nov. 12, 2014, and the picture after that shows the bronze and plastic knots next to each other for comparison of size and color.
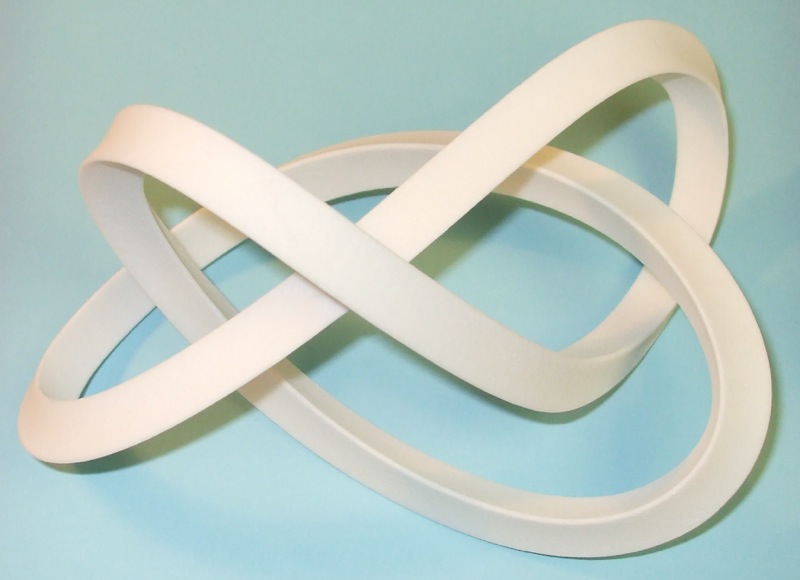

When I showed my colleague, Marcin Mazur, the large plastic Mobius Figure 8 Knot, he suggested that it would be interesting if a marble could roll around the single-sided surface. After a little thought, I realized that a modified cross section could achieve that goal. I replaced the concave triangle cross section with a circle with three smaller circles removed so that the resulting groove goes around the knot three times before returning to its starting point. A 1/4" diameter steel ball bearing inserted into the groove would be held in because the diameter of the ball is slightly larger than the opening of the groove. The first version I had printed by Shapeways did not work well because I only made the groove .005" larger than the diameter of the ball, and the ball would not freely roll around the groove. I redesigned it with a larger groove (.015" larger than the diameter of the ball), and the result is shown in the following picture. The ball is easily pushed into the groove, since the plastic is flexible, and stays in the groove all around except at one sharp curve, where the opening is too wide. So I think one more design adjustment will make it perfect. I call it the ``Groovy Mobius Figure 8 Knot".

As shown on the webpage Sculptures from Spring 2014, I made a bronze version of the logo for the IHES. I also decided to try to design a lapel pin version of it, and the following picture shows how it came out 3D printed by Shapeways in stainless steel. The pin on the back works fine with a standard pin clip, but a plastic version I also had printed was too fragile, and would have to be made thicker for the pin to work.

As shown on the webpage Sculptures from Spring 2007, I made a bronze sculpture inspired by DNA strands, with a pair of curves spiraling in towards each other as they rise to a common point, twisting around a central axis, each surface having as cross section a hypocycloid. I decided to try to make a design like that which could be 3D printed. But the size limitations (at most 15 cm tall) and the limitations of the strength of the material (strong flexible white plastic), meant that I had to make the points at the top meet and be wide enough to pass the Shapeways inspection so they would not break during polishing. My first version did not pass inspection, so I redesigned it, and on Dec. 22, 2014, received a 3D printed version whose pictures are below. These two pictures show it from the side and from above.


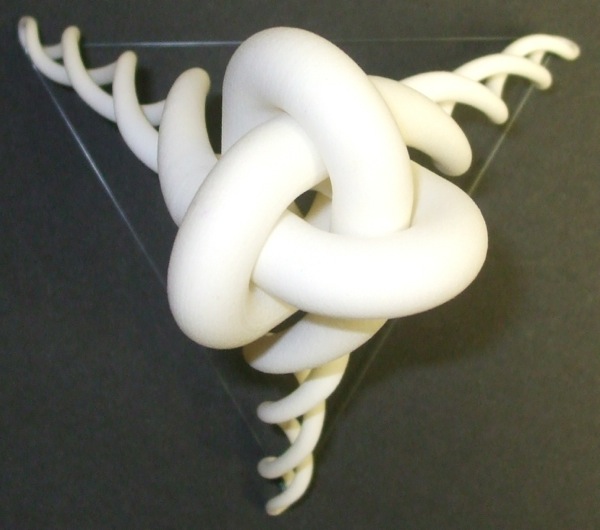
As shown on the webpage Sculptures from Spring 2006, I made a bronze sculpture inspired by the Borromean rings, and by Helaman Ferguson's twisted realization of them. Below are two pictures (front and top views) of my interpretation of it 3D printed by Shapeways. In order to have it stand up higher, I tied the base points with fishing line (like a tensegrity). Without tying, the three pieces are loose and it stands quite lower. The tripod stands almost 6" tall, and with the bottom points tied, the triangle made by the feet is about 5" on a side.

As shown on the webpage Sculptures from Summer 2007, I made bronze Borromean rings (with patina). It was not hard to put the design into a computer model, and below are two pictures of it 3D printed by Shapeways. The size of each link is about 2.5".


I had another copy of the large Mobius Figure 8 Knot printed by Shapeways, to try and get a more uniform surface. Sometimes the printing process leaves lines which were not in the design. Here is a picture of the best one they have made for me so far. It is almost 6" in the longest direction.

I have opened a ``Shapeways Shop", Alex Feingold's Mathematical Art, where some of my designs are now available for purchase as 3D printed objects in various materials (strong flexible plastics in various colors, stainless steel, bronze, silver, etc.). As I perfect more designs, I may make more of them available for sale there. The responsibility for these projects is entirely mine, and I am only using my Binghamton University website to provide information about their commercial availability in the same way that I might provide information about the availability of a textbook or other useful educational resource.
To see more types of sculpture I have tried, follow the following links:
Links back to:
Webpage of Alex Feingold,
Department of Mathematical Sciences,
Binghamton University.